The Math Dynamics Multivariable Algebra Expression Based Graphing Calculator

Complete the Square method is an algorithm to find the Roots of a Quadratic Equation both Real and Complex. This exercise focuses on using the “Complete the Square” method to calculate the Real Roots so it only makes sense to use a Quadratic Function that has two real roots.
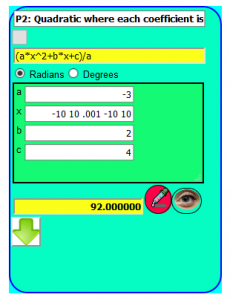
We start with a Quadratic Function defined as:
\( -3*x^2 + 2*x + 4 \)
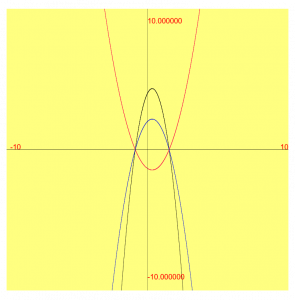
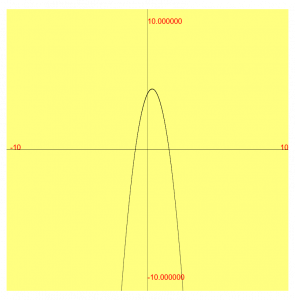
The plot of this function visually demonstrates that this Quadratic Function has 2 real roots.
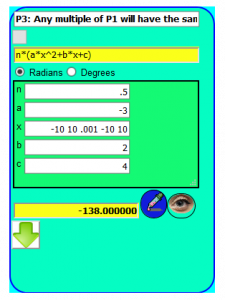
The exact values of these two real roots can be calculated using the “Complete the Square” method. This requires two things:
- \( -3*x^2 + 2*x + 4 = 0\)
- The \(a*x^2\) term requires that a = 1, not -3
To resolve the second requirement requires that both sides of the quadratic equation be multiplied by -(1/3) such that the new Quadratic Function is:
\( x^2 -(2/3)*x -(4/3) = 0\)
Which produces a different graph. This new graph shares the same roots as the original graph.

I found this to be a quite interesting property. It appears though that all multiples of a Quadratic Function have equal roots